15.6 Longitudinal APIM
Longitudinal variants of the APIM are some of the most interesting dyadic models. Here we will consider some aspects of the longitudinal APIM.
15.6.1 Model
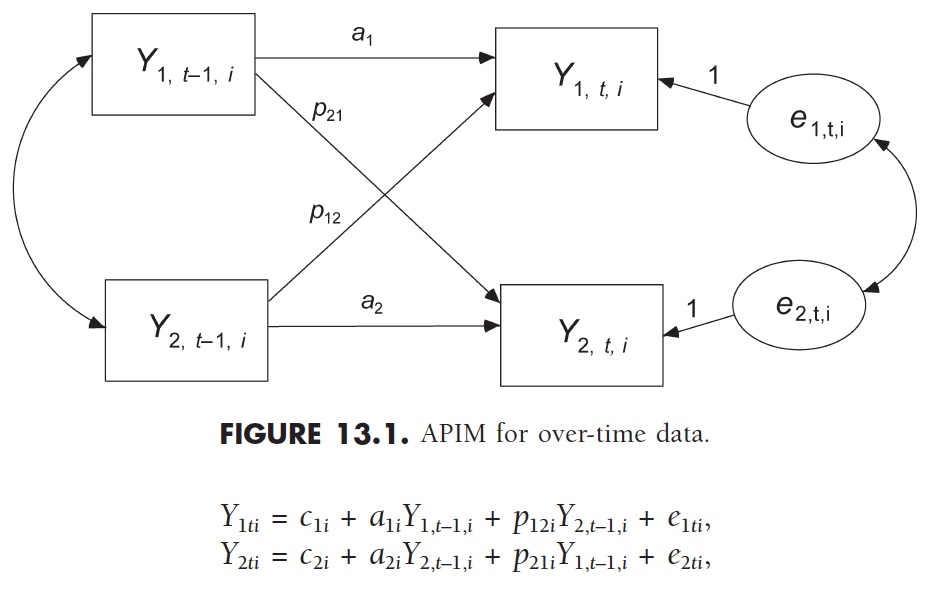
In the longitudinal APIM,
- actor effects are the effects from \(Y_{1,t–1}\) to \(Y_{1,t}\) (\(a_{1i}\)) and from \(Y_{2,t–1}\) to \(Y_{2,t}\) (\(a_{2i}\)),
- actor effects are interpreted as stability effects
- partner effects are from \(Y_{1,t–1}\) to \(Y_{2,t}\) (\(p_{21i}\)) and from \(Y_{2,t–1}\) to \(Y_{1,t}\) (\(p_{12i}\)),
- partner effects represent cross-partner influence, or reciprocity
15.6.2 Estimated Parameters

Typically in the longitudinal APIM we are interested in estimating the following parameters for each of the \(i\) dayds:
- Actor effect for person 1, or \(a_{1i}\).
- Actor effect for person 2, or \(a_{2i}\).
- Partner effect from person 1 to person 2, or \(p_{12i}\).
- Partner effect from person 2 to person 1, or \(p_{21i}\).
- Intercept for person 1, or \(c_{1i}\).
- Intercept for person 2, or \(c_{2i}\).
Each of these parameters may also vary across dyads.
For example, if \(a_{1}\) (the average value of dyad member 1’s stability value) varies, then for some 1’s, there is more stability than there is for other 1’s.
Similarly, there might be variance in the partner effects such that individuals in some dyads may be more responsive or reactive to their partners than are individuals in other dyads.
15.6.3 Parameter Covariation
These six terms (two actor, two partner, and two intercepts) may also covary with each other. It is helpful to consider some of these correlations and how we might interpret them.
Correlation between intercepts
Suppose we measure stress each day for a parent-child dyad. The correlation between the intercepts would measure the extent to which parents who experience more stress overall have children who also experience more stress overall. Thus the unit for the correlation of intercepts is the person.
Correlation between errors
Let’ say a parent experiences more stress on a particular day. More stress than one would expect given their prior value and their child’s prior value, A positive correlation between the errors would indicate that is likely the child would also experience heightened stress on that day.